Another Example of MOAs using Ratio of Means or Difference of Means

You direct the medical records unit in a medium-sized hospital.   You are concerned about the number of errors that are made in the unit when filing/coding diagnoses and procedures.   In order to determine the best corrective action to take, you collect information for each of your employees on the number of filing/coding errors made in the last month, whether the employee is a Registered Health Information Administrator (RHIA) or Registered Health Information Technician (RHIT), and the number of years of experience in filing/coding.  

Your data are below in Table 2.  

TABLE 2: Filing errors last month, RHIA/RHIT status, and years of experience for 12 medical record employees, Wilted Lilly Hospital, June, 2013.

         

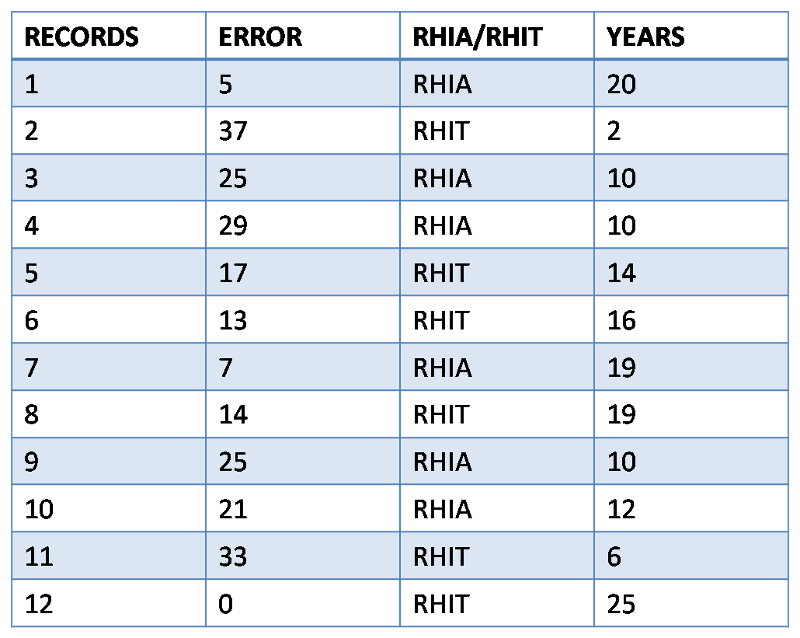
ERROR=number of filing/coding errors in month (per 1000 records), RHIA/RHIT=certification held, YEARS=years of filing experience.

In order to test if certification and years of experiences are potential causes for coding errors, we have to:

1. Evaluate the relationship between certification (RHIA/RHIT) and filing errors.
2. Evaluate the relationship between years of experience and filing errors.

For Task #1, What kinds of MOAs should we use here? Well, Certification is a nominal variable while filing errors is a quantitative variable, so we should use Difference in Means (or medians) or Ratio of Means (or medians)

For Task #2, What kinds of MOAs should we use here? Well, Years of experience is a quantitative variable and filing errors is a quantitative variable, we can't use DM, RM, RR, AR, or AP. We will have to use a new MOAs called regression coefficient or slope and we will discuss it in detail later in this lesson.

Now Let's complete Task #1. Remember we need to examine difference in means (or medians) or ratio of means(or medians), so we first we need to find out mean and median filing error for each certification.

For RHIA certification, filing errors (per 1000 records) are: 5, 25, 29, 7, 25, 21; mean =18.7; median = 23

For RHIT certification, filing errors (per 1000 records) are: 37, 17, 13, 14, 33, 0; mean = 19; median = 15.5

Notice mean and median for each certification are quite different (18.7 vs 23; 19 vs 15.5), indicating a skewed distribution. Remember in Lesson 5, we have discussed that for skewed distribution, median is a better measure to use than mean? So in this case, we should use Difference in Median or Ratio of Median and presented following the format of Table 1.

Note the group of RHIT is used as the reference group.

[1]: 23/15.5 = 1.48

Interpretation: The average filing errors made by employees with RHIA certification is 1.48 times as high as (or 48% higher than) that by employees with RHIT certification.

[2]: 23-15.5 = 7.5

Interpretation: The average filing errors made by employee with RHIA certification is 7.5 errors (per 1000 records) more than that by employee with RHIT certification.

Based on above results, we can see that types of certification one receives do appear to be associated with filing errors.

Another important point to keep in mind is that Difference of Means and Ratio of Means can be replaced with Difference of Medians or Ratio of Medians depending on the distribution of the quantitative variable.

Have I Grasped the Key Concepts Here?

To examine the potential causes of filing errors, data on the number of filing errors (per 1000 records) made in the last month, whether the employee is a RHIA or RHIT, and the number of years of experience in coding are collected and presented below:

Based on the above table, answer the following questions:

Assuming the following results are obtained after analyzing the above data (Note RHIT is used as the reference group):

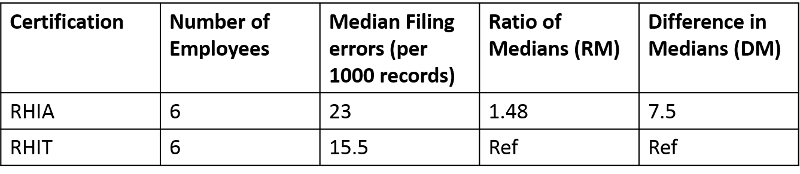
Based on the above table, answer the following questions: